|
|
 发表于 28-10-2009 03:33 PM
|
显示全部楼层
发表于 28-10-2009 03:33 PM
|
显示全部楼层
你们好。。我这里有一题关于matrices的问题。。
我不是很了解题目要的是什么。。所以请你们帮帮忙。。谢谢。。
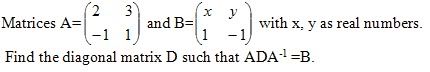 |
|
|
|
|
|
|
|
|
|
|
|
 发表于 28-10-2009 04:06 PM
|
显示全部楼层
发表于 28-10-2009 04:06 PM
|
显示全部楼层
回复 481# bell_25 的帖子
D is diagonal matirx :
(a 0
0 b)
ADA^-1 = B [x A^-1]
AD=BA
then find unknown a & b |
|
|
|
|
|
|
|
|
|
|
|
 发表于 28-10-2009 04:19 PM
|
显示全部楼层
发表于 28-10-2009 04:19 PM
|
显示全部楼层
[quote]原帖由 Allison 于 28-10-2009 04:06 PM 发表 
D is diagonal matirx :
(a 0
0 b)
ADA^-1 = B [x A^-1]
AD=BA
× A |
|
|
|
|
|
|
|
|
|
|
|
 发表于 28-10-2009 04:37 PM
|
显示全部楼层
发表于 28-10-2009 04:37 PM
|
显示全部楼层
回复 482# Allison 和 483# Log 的帖子
谢谢你们两位。。意思是说直接找a和b就好了。。
x和y可以不理..
我找到的答案是 a=-1 和 b=-4
然后再拿a和b的value来form matices D。。
对吧? |
|
|
|
|
|
|
|
|
|
|
|
 发表于 28-10-2009 10:43 PM
|
显示全部楼层
发表于 28-10-2009 10:43 PM
|
显示全部楼层
回复 484# bell_25 的帖子
errm....
a=-3, b=2
 |
|
|
|
|
|
|
|
|
|
|
|
 发表于 29-10-2009 12:03 AM
|
显示全部楼层
发表于 29-10-2009 12:03 AM
|
显示全部楼层
回复 485# Log 的帖子
哦。。不好意思。。我做错了。。
哈哈。。谢谢你的提点。。 |
|
|
|
|
|
|
|
|
|
|
|
 发表于 29-10-2009 02:09 PM
|
显示全部楼层
发表于 29-10-2009 02:09 PM
|
显示全部楼层
回复 483# Log 的帖子
不好意思。。。我写错了 |
|
|
|
|
|
|
|
|
|
|
|
 发表于 2-11-2009 05:05 PM
|
显示全部楼层
发表于 2-11-2009 05:05 PM
|
显示全部楼层
嗨。。我又有问题要发问咯。。
希望你们帮帮我。。谢谢
∫ [ 1 / (sinx cosx) ] dx
要怎样solve这题呢? |
|
|
|
|
|
|
|
|
|
|
|
 发表于 2-11-2009 05:13 PM
|
显示全部楼层
发表于 2-11-2009 05:13 PM
|
显示全部楼层
原帖由 bell_25 于 2-11-2009 05:05 PM 发表 
嗨。。我又有问题要发问咯。。
希望你们帮帮我。。谢谢
∫ [ 1 / (sinx cosx) ] dx
要怎样solve这题呢?
上下乘2, 把它变成2/ (sin2x), 然后才integrate |
|
|
|
|
|
|
|
|
|
|
|
 发表于 2-11-2009 06:23 PM
|
显示全部楼层
发表于 2-11-2009 06:23 PM
|
显示全部楼层
回复 488# bell_25 的帖子
by using substitution ,
let u=sin x , du/dx=cos,
then solve..... |
|
|
|
|
|
|
|
|
|
|
|
 发表于 3-11-2009 09:32 PM
|
显示全部楼层
发表于 3-11-2009 09:32 PM
|
显示全部楼层
(a) f(1+x^3)=5x^2-10 , FIND f ' (9)..........the answer is 5/3
(b) 100^x - 10^ (x+1) + k = 0 ...............the answer is 25> k >0
how to do this 2 question? anyone can help me? please and thank you... |
|
|
|
|
|
|
|
|
|
|
|
 发表于 4-11-2009 12:04 AM
|
显示全部楼层
发表于 4-11-2009 12:04 AM
|
显示全部楼层
回复 489# cgcy & 490# Log 的帖子
谢谢你们。。我知道怎样做了。。 |
|
|
|
|
|
|
|
|
|
|
|
 发表于 4-11-2009 12:25 AM
|
显示全部楼层
发表于 4-11-2009 12:25 AM
|
显示全部楼层
原帖由 星星之玲 于 3-11-2009 09:32 PM 发表 
(a) f(1+x^3)=5x^2-10 , FIND f ' (9)..........the answer is 5/3
(b) 100^x - 10^ (x+1) + k = 0 ...............the answer is 25> k >0
how to do this 2 question? anyone can help me? please a ...
(b)的问题似乎不完整。。我不明白题目要什么。。所以无法给你帮上忙。。
for question (a) 我的方法是。。
f(1+ x^3) = 5x^2 - 10
let u = 1 + x^3
x^3 = u - 1
x^2 = (u - 1)^(2/3)
f(u) = 5[(u - 1)^(2/3)] - 10
f '(u) = (10/3) [(u - 1)^(-1/3)]
f '(1+ x^3) = (10/3) [(1 + (x^3) - 1)^(-1/3)] = (10/3) ( x^-1)
1+ x^3 = 9
x^3 = 8
x= 2
f '(9) = (10/3)(2^-1) = 5/3 |
|
|
|
|
|
|
|
|
|
|
|
 发表于 4-11-2009 10:45 AM
|
显示全部楼层
发表于 4-11-2009 10:45 AM
|
显示全部楼层
100^x - 10^(x+1) + k = 0
(10^x)^2 - (10)(10^x) + k = 0
let 10^x = y
y^2 - 10y + k = 0
Using b^2 - 4ac > 0 , since 10^x =/= 0 for all value of x.
100 - 4(1)(k) > 0
k < 25
when x -----> -inifnity , 10^x -------> 0
thus, (0)+10(0)+k = 0
k = 0 ( is the smallest value of k )
thus k={k= IR; 0 < k < 25}
dunno correct or not but pls check if any wrong on my answer. |
|
|
|
|
|
|
|
|
|
|
|

楼主 |
发表于 4-11-2009 01:58 PM
|
显示全部楼层
2y=a^x + a^-x
prove a^x= y + square root of ( y^2 - 1 ) |
|
|
|
|
|
|
|
|
|
|
|
 发表于 4-11-2009 02:43 PM
|
显示全部楼层
发表于 4-11-2009 02:43 PM
|
显示全部楼层
回复 495# 白羊座aries 的帖子
hints: let u= a^x,then form a quadratic equation and find the root using。。。。。quadratic formula,
so get it |
|
|
|
|
|
|
|
|
|
|
|

楼主 |
发表于 4-11-2009 03:58 PM
|
显示全部楼层
|
|
|
|
|
|
|
|
|
|
|
 发表于 4-11-2009 04:15 PM
|
显示全部楼层
发表于 4-11-2009 04:15 PM
|
显示全部楼层
回复 497# 白羊座aries 的帖子
then, 2y±√4(y²-1) / 2= 2y ± 2√(y²-1)/ 2
ok?
[ 本帖最后由 Log 于 4-11-2009 04:18 PM 编辑 ] |
|
|
|
|
|
|
|
|
|
|
|

楼主 |
发表于 4-11-2009 05:35 PM
|
显示全部楼层
|
|
|
|
|
|
|
|
|
|
|
 发表于 4-11-2009 11:37 PM
|
显示全部楼层
发表于 4-11-2009 11:37 PM
|
显示全部楼层
回复 499# 白羊座aries 的帖子
not at all |
|
|
|
|
|
|
|
|
|
| |
 本周最热论坛帖子 本周最热论坛帖子
|